
Home
Common Compounds
Exam Guide
FAQ
Features
Glossary
Construction Kits
Companion Notes
Just Ask Antoine!
Slide Index
Toolbox
Tutorial Index
Companion Notes
Introduction
Measurement
Matter
Atoms & ions
Compounds
| Chemical change |
Gases
Energy & change
The quantum theory
Electrons in atoms
The periodic table
Home  Companion Notes Companion Notes  Chemical change Chemical change | Print | Comment |

- Always check the equation by counting atoms on each side of the equation. For each element, the number of atoms on the left has to be equal to the number of atoms on the right.
H2SO4(aq) + Ba(OH)2(aq) 
2 H2O(l) + Na2SO4(aq) 4 hydrogens, 1 sulfur, 1 barium, 6 oxygens 4 hydrogens, 1 sulfur, 1 barium, 6 oxygens 
2(NH4)3PO4(aq) + 3 CaCl2 
Ca3(PO4)2(s) + 6 NH4Cl(aq) 24 hydrogens, 6 nitrogens, 2 phosphoruses, 8 oxygens, 3 calciums, 6 chlorines 24 hydrogens, 6 nitrogens, 2 phosphoruses, 8 oxygens, 3 calciums, 6 chlorines - Start by putting a "1" in front of the most complex compound (the one containing the greatest number and variety of atoms).
1 C6H12O6(s) + O2(g)
 CO2(g) + H2O(l)
CO2(g) + H2O(l)
- Balance elements that appear in multiple reactants or multiple products last. In combustion equations, this means that the oxygen is always balanced last.
...in the equation above, balance the O last, because it appears in more than one compound on each side of the equation.
Balancing C's: 1 C6H12O6(s) + O2(g)
 6 CO2(g) + H2O(l)
6 CO2(g) + H2O(l)
Balancing H's: 1 C6H12O6(s) + O2(g) 6 CO2(g) + 6 H2O(l)
6 CO2(g) + 6 H2O(l)
Balancing O's: 1 C6H12O6(s) + 6 O2(g) 6 CO2(g) + 6 H2O(l)
6 CO2(g) + 6 H2O(l)
- Whole number coefficients are preferred. But use fractions during the balancing process. The equation can be scaled to remove them later.
Try a "1" for most
complex compound1 C3H7S(l) + O2(g)  CO2 + H2O(l) + SO2
CO2 + H2O(l) + SO2
Balancing C's: 1 C3H7S(l) + O2(g)  3 CO2 + H2O(l) + SO2
3 CO2 + H2O(l) + SO2
Balancing S's: 1 C3H7S(l) + O2(g)  3 CO2 + H2O(l) + 1 SO2
3 CO2 + H2O(l) + 1 SO2
Balancing H's: 1 C3H7S(l) + O2(g)  3 CO2 + 7/2 H2O(l) + 1 SO2
3 CO2 + 7/2 H2O(l) + 1 SO2
2 C3H7S(l) + O2(g)  6 CO2 + 7 H2O(l) + 2 SO2
6 CO2 + 7 H2O(l) + 2 SO2
Balancing O's: 2 C3H7S(l) + 23/2 O2(g)  6 CO2 + 7 H2O(l) + 2 SO2
6 CO2 + 7 H2O(l) + 2 SO2
4 C3H7S(l) + 23 O2(g)  12 CO2 + 14 H2O(l) + 4 SO2
12 CO2 + 14 H2O(l) + 4 SO2
- Be certain that the total charge is the same on each side after you have a mass-balanced equation.
Mass balanced but not charge balanced: Zn(s) + Fe3+(aq)  Zn2+(aq) + Fe2+(aq)
Zn2+(aq) + Fe2+(aq)
Both mass and charge balanced Zn(s) + 2 Fe3+(aq)  Zn2+(aq) + 2 Fe2+(aq)
Zn2+(aq) + 2 Fe2+(aq)
- Don't touch the subscripts! Changing the subscripts in a formula results in an entirely different chemical compound (and often one that doesn't exist). Remember that the coefficients show how much compound is involved in the reaction, while the subscripts show how much element is in the compound.
NaOH(aq) + Al2(SO4)3(aq)
 Al(OH)3(s) + Na2SO4(aq)
Al(OH)3(s) + Na2SO4(aq)
Wrong: Na2(OH)3(aq) + Al2(SO4)3(aq) Al(OH)3(s) + Na2(SO4)3(aq)
Al(OH)3(s) + Na2(SO4)3(aq)
Correct: 6 NaOH(aq) + Al2(SO4)3(aq) 2 Al(OH)3(s) + 3 Na2SO4(aq)
2 Al(OH)3(s) + 3 Na2SO4(aq)
- When you notice that the same polyatomic ions appear on both sides of the equation, it's much easier to balance the equation as though the polyatomic ions were 'atoms'. For example:
2(NH4)3PO4(aq) + 3 CaCl2 
Ca3(PO4)2(s) + 6 NH4Cl(aq) 24 hydrogens, 6 nitrogens, 2 phosphoruses, 8 oxygens, 3 calciums, 6 chlorines 24 hydrogens, 6 nitrogens, 2 phosphoruses, 8 oxygens, 3 calciums, 6 chlorines 6 ammoniums, 2 phosphates, 3 calciums, 6 chlorides 6 ammoniums, 2 phosphates, 3 calciums, 6 chlorides - A missing coefficient is understood to be a "1".
1 HCl(aq) + 1 NaOH
 1 NaCl(aq) + 1 H2O(l)
1 NaCl(aq) + 1 H2O(l)
is more compactly written as
HCl(aq) + NaOH NaCl(aq) + H2O(l)
NaCl(aq) + H2O(l)
but both are technically correct! - Write the equation so that the coefficients are the smallest set of integers possible. For example, if all of the coefficients are divisible by 2, divide them all by 2.
2 H3O+(aq) + 2 OH-
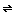 4 H2O(l)
4 H2O(l)
is more compactly written as
H3O+(aq) + OH-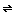 2 H2O(l)
2 H2O(l)
but both are technically correct! - Equations that involve electron transfer (redox reactions) are sometimes difficult to balance by inspection.
When you notice that the oxidation numbers for an element aren't identical on both sides of the equation, you have a redox equation. You can sometimes balance such equations by inspection if the equation is simple (and if you're stubborn enough) but there is a special recipe for balancing redox equations that relies on balancing the changes in oxidation number. We'll show you the recipe in second semester general chemistry, when redox reactions are discussed in more detail.
If you're ready for the recipe now, here's a sketchy outline of the procedure for balancing redox reactions:
- Assign oxidation numbers to atoms in all species.
- Identify which reactant is being oxidized (the oxidation number increases when it reacts) and which is being reduced (the oxidation number goes down).
- Write the reaction as two separate "half reactions": one for the oxidation, and one for the reduction.
- Balance each separate half reaction as follows:
- Balance missing oxygens by adding H2O to the opposite side the equation.
- Balance missing hydrogens by adding H+ to the opposite side.
- Balance the charge by adding electrons (remembering that oxidation involves electron loss, and reduction involves electron gain).
- Multiply each half reaction by an integer so that the number of electrons in both half reactions is the same.
- Add the two half reactions together.
- If the reaction is taking place in alkaline solution, and the resulting equation contains H+, add the reaction for the dissociation of water
H2O(l)
to the equation to eliminate hydrogen ions.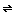 H+(aq) + OH-(aq)
H+(aq) + OH-(aq)
newsletter describing updates,
new features, and changes
on this site.
General Chemistry Online! Balancing equations
Copyright © 1997-2005 by Fred Senese
Comments & questions to fsenese@frostburg.edu
Last Revised 02/23/18.URL: http://antoine.frostburg.edu/chem/senese/101/reactions/balancing.shtml